#Jordan curve theorem

[mi-luli]

[its-a-hil]

i know something has gone horribly wrong when im looking up a math thing and see a link leading to the jordan curve theorem. absolutely not. worst thing in mathematics

[jimathanscrimbly]

the jordan curve theorem says that every simple closed curve divides a plane into an inside and an outside

[max1461]

“yeah my name’s jordan, like the curve theorem”

[transgenderer]

when i learned the jordan curve theorem was proved by someone named "camille jordan" i was like, oh cool 19th century female mathematician, but no "Marie Ennemond Camille Jordan" is a man

[canadianfruitpunch]

differential geometry prof: the proof for the Jordan curve theorem is surprisingly difficult but it’s a fun exercise if you have the time for it but you’ll need a grad level algebraic topology text for reference

also differential geometry prof, one slide later: remember the circumference of a circle is 2pi*r

[relevant-wikipedia-articles]

I love when a textbook has a theorem that is just the most obviously true shit, like girl I sure hope so, if that weren't true I think math would fall apart!

[seeselfblack]

Happy BornDay Dudley Weldon Woodard

Dudley Weldon Woodard was born on this date in 1881. He was a Black mathematician.

From Galveston Texas his father worked for the U. S. Postal Service. Woodard was a smart youngster whose curiosity was supported by his family. After finishing his primary education in his home state, Woodard attended Wilberforce College in Ohio, receiving a bachelor degree (A. B.) in mathematics in 1903. He then received a B. S. degree in 1906 and an M. S. degree in mathematics at the University of Chicago in 1907. From 1907 to 1914, Woodard taught mathematics at Tuskegee Institute and then moved to join the Wilberforce faculty from 1914-1920.

In 1921, he joined the mathematics faculty at Howard University. During this time received his Ph.D. Mathematics in 1928 at the University of Pennsylvania. Woodward’s thesis was entitled: On Two-Dimensional Analysis Situs with Special Reference to the Jordan Curve Theorem. While at Howard, he was also selected Dean of the College of Arts and Sciences where he worked until 1929. Woodard became the second Black to earn a Ph.D. degree in mathematics. Woodard was married and had a son who later joined the faculty at Howard University. Dr. Woodard established the M. S. degree program in mathematics, making Howard University's mathematical program as one of the best for study among America’s Historically Black Universities and Colleges (HBCU).

He was the thesis supervisor for many of Howard's M. S. degree students. He also established the mathematics library at Howard. He established and sponsored several professorships and many scholarly seminars in mathematics. Among his colleagues and students, Woodard excelled and was very popular as professor and administrator. He was highly respected by those who knew him in the mathematical sciences community. Deane Montgomery, former president of the American Mathematical Society and the International Mathematical Union described Woodard as, "an extremely nice man, well-balanced personally." Leo Zippin, who was an internationally known specialist in Woodard's field, said that he was "one of the noblest men I've ever known."

Dr. Woodard was not only a brilliant mathematician, but a man of dignity; he enjoyed life in spite of his racial environment. He used the phrase, "black is beautiful" in the 1930s; he often ignored the "colored" signs and visited any men's restroom of his choice.  He also ate at many "nice" restaurants and enjoyed the theaters of his choice in New York. He and his family once moved in what had been an all-white neighborhood because it was aesthetically nice and it was near Howard. Woodard devoted his entire professional life to the promotion of excellence in mathematics through the advancement of his students, teaching and research. Dudley Woodard retired in 1947 and died July 1, 1965 in his home in Cleveland Ohio.

Reference: - The Modern History of Blacks in Mathematics

- To Become A Mathematician

See Also: 

- 8 Famous Black Mathematicians and Their Contributions 

[lthmath]

A Little History of Calculus

In the middle of the 19th century Riemann introduced his theory of integration. The last third of the century saw the arithmetization of analysis by Weierstrass, who thought that geometric reasoning was inherently misleading, and introduced the "epsilon-delta" definition of limit. Then, mathematicians started worrying that they were assuming the existence of a continuum of real numbers without proof. Dedekind then constructed the real numbers by Dedekind cuts, in which irrational numbers are formally defined, which serve to fill the "gaps" between rational numbers, thereby creating a complete set: the continuum of real numbers, which had already been developed by Simon Stevin in terms of decimal expansions. Around that time, the attempts to refine the theorems of Riemann integration led to the study of the "size" of the set of discontinuities of real functions.

Also, "monsters" (nowhere continuous functions, continuous but nowhere differentiable functions, space-filling curves) began to be investigated. In this context, Jordan developed his theory of measure, Cantor developed what is now called naive set theory, and Baire proved the Baire category theorem. In the early 20th century, calculus was formalized using an axiomatic set theory. Lebesgue solved the problem of measure, and Hilbert introduced Hilbert spaces to solve integral equations. The idea of normed vector space was in the air, and in the 1920s Banach created functional analysis.

[ambivalencerelations]

Learn Complex Analysis

On your own!

The math behind it:

The limit definition of a derivative

Mathematical definitions of continuity and convergence

Convergence tests

Integration, Fundamental Theorem of Calculus, integration tricks and multi variable integration

Green's Theorem and identities

Partial derivatives

Harmonic functions and why they are cool

Complex numbers and arithmetic

Euiler's identity

Taylor series and radii of convergence

Some R^2 related set theory terms like "open", "neighborhood", "domain", and "bounded"

Curve related terms like Jordan, simple, closed, and k-connected

With those, you could then understand actual complex analysis:

What analytic functions are and why we care

Notation like f, F, z, x, y, omega, gamma, capital gamma, alpha, theta, r, epsilon

Ways to think about analytic functions that are useful, like splitting them up, in terms of polar co-ordinates, etc.

The Cauchy-Riemann Equations (duh)

The link between Harmonicity and Analyticity, harmonic conjugates.

Sturm and louiville theorems

Maximum modulus principle, minimum modulus, strong/weak variations

The ML inequality

Integration in complex numbers

Playing with du, dz, d$\alpha$ and dF

Cauchy Integral theorem

Cauchy Integral formula (Amazing!)

Cauchy Integral formula for higher derivatives of f (EVEN BETTER!)

Analyticity implies infinite derivatives

Analyticity implies many nice things

Cauchy convergence criterion

Uniform convergence (UC)

Weirstrass M test

If a sequence of continuous, differentiable, or analytic functions UC, then it is to a continuous, differentiable, or analytic function.

Absolute convergence means you can fiddle with the order of the terms and other things

Analytic functions yeild power series

Power series yeild Analytic functions

Analyticity and Taylor series

Removable discontinuities/poles

Residue theorem!!!!

???

Profit

I also highly suggest the cheap, highly readable textbook: "Complex Variables: Harmonic and Analytic Functions" by Francis J Flanigan.

[deaths-accountant]

you don't need the Jordan Curve Theorem, you just need the change in angle at that vertex, so you can check each vertex has the same change in angle- which is tau/4 or 3tau/4.

We should have shoved nerds into lockers HARDER.

[mathblab]

Mathematics is one of the essential emanations of the human spirit—a thing to be valued in and for itself like art or poetry.

Oswald Veblen, mathematician known for proving the Jordan curve theorem, a theorem thought to be so obvious that no one bothered proving it until Veblen in 1905 https://people.math.osu.edu/fiedorowicz.1/math655/Jordan.html

[mathematicianadda]

Jordan Curve Theorem, Professor Tao's proof

Here is Professor Terry Tao's proof of the Jordan curve theorem using complex analysis, I more or less followed the proof until the following paragraph (see section 4). (Actually there is no need to read everything before the following paragraph to answer the question.)

https://terrytao.wordpress.com/2016/10/02/math-246a-notes-3-cauchys-theorem-and-its-consequences/

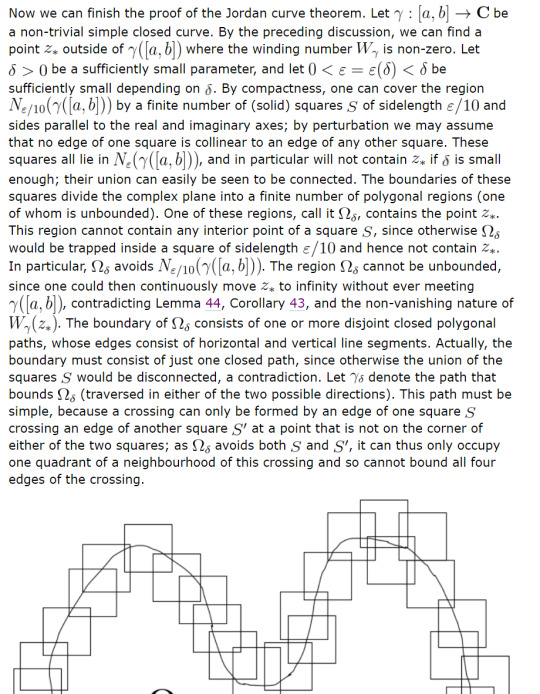
There are two things which I do not understand:

firstly, how do we know the boundary of $\Omega_\delta$ consists of one or more simple closed curves? How to rigorously demonstrate that its boundary cannot be some random collection of segments? Also, why does the fact that the union of squares is connected implies the boundary of $\Omega_\delta$ consists of exactly one simple closed curve. Also the sentence on why the boundary of $\Omega_\delta$ is simple got me confused.(last sentence of the paragraph above the picture)

secondly, what's the point of covering $N_{\epsilon/10}(\gamma([a,b]))$? Why not just cover $\gamma([a,b])$?

Any explanation would be immensely appreciated!!

Here $W$ denotes the winding number. $N_{\epsilon/10}(\gamma([a,b]))$ is the set of points $z\in\mathbb{C}$ such that $\text{distance}(z,\gamma)<\epsilon/10.$

from Hot Weekly Questions - Mathematics Stack Exchange from Blogger https://ift.tt/2WRgPBT

[nerdastically]

Hello ^^ I'm interested in coding, but it's still very, very much entry level in regards to experience ^^' i was wondering what kind of math computer science goes up to... and this might be a dumb question, but how is math (I'm talking like calculus or geometry) involved with computer science? Also, how are classes? Exams? Assignments? Thank you!

Hi there  ✨ Math in computer science helps you better understand how the computers process data, how they calculate. Let’s say you have to program a calculator that also draws graphs. You have to know what the output will be so you can code it properly. But that’s a very narrow example. Math can be also used to calculate for example (in 3D graphics, designing a game) a path of the object’s movement like if the object is to move along a certain curve (they never move truly randomly) you ought to type in an equation of that curve. Well, it’s mostly that kind of stuff. Plus math makes you think and this is the same kind of thinking you’re using while coding. 

I’d say we had a high level of math like it was difficult and many people didn’t pass it right away. The hardest during the first semester was calculus because we haven’t had that in school, but since you have it it should be easy for you. We covered a lot of advanced proofs and theorems (eg. Taylor’s theorem, Cauchy condensation and so on). Then there was linear algebra and geometry. That was quite hard as well. We did Jordan normal form, but the geometry wasn’t that difficult!  ✨ Other than that, math subjects (differential equations, statistics or logic, and estimation) were rather manageable, they taught us everything step by step. Calculus and Algebra were the only subjects that you had to do pretty much all the work at home, by yourself. That’s probably why most people didn’t pass that. As long as you’re fine with working on your own, you should be okay. 

Calculus exam was hell. We had 2.5h and 5 tasks which were tricky and you had to read very carefully. But I bet if you studied hard during the year (and not just before the exam like I did lmao), it was ok! 

Algebra exam was kind of hard, but not as hard as calculus. And the professor told us everything that will be on the exam. We had 3 tasks and 1.5h. (2 algebra, 1 geometry)

We didn’t really have any assignments. They just assumed we will study every topic after the class and you really should do that if you want to be well prepared for the exam. We had 2 tests during the classes (both calculus and algebra) & 1 big final exam. I failed the first test on calculus, but it was ok because we had to have 50% from the sum of both tests (it wouldn’t work on algebra). 

Well, idk how it will be for you! I’ve basically told you how it’s done here, but you know, it might be different for where you live. Maybe you should ask around on some uni’s online chats or fb group? :) The syllabus might differ a bit! 

[locally-normal]

I've forgotten this out loud on this bog before but I think the limit is a counterexample to the generalized Jordan curve theorem. It's homeomorphic to a sphere, and when you embed it in euclidean space as shown, the inside is homeomorphic to a disk, but the exterior isn't homeomorphic to the exterior of the standard embedding of a disk.

So, not strictly a conjecture about limits, really just using a limit to build a counterexample to some other reasonable conjecture.

Also, you can't upgrade this to diffeomorphisms (or even piecewise linear) so it was an early demonstration of the differences between the categories.

Screaming because my friend the Alexander Horned Sphere made it into a differential geometry book

[max1461]

Iraq my brain (easy)

Iran away (easy)

Yeah, I Kuwait a minute (easy)

Oman, that was crazy (easy)

I know you don't believe me, but I swear it Isreal (easy)

Micheal Jordan (alt: Jordan curve theorem) (easy)

Yemen, I totally agree (easy but I did have to think a minute)

My friend asked me to pass him his large German beer mug, so I said ok and gave my Palestine (medium)

Wow, looks like we Qatar selves a big fish (medium)

Would you like me to Syria a steak? (medium)

Me: "I'll have the Unagi please." Waiter: "Ok, I'll bring UAE-l" (medium, also bad)

As a music producer working with many famous clients, I gave a ceLebenon-negotiable contract (hard)

My colleague Beatrice at the SCP foundation who I'm secretly having a romance with: "those data structures... they encode memetic hazards. Anyone who looks at them will instantly drop dead after 24 hours." Me, not sure what to say as the realization sets in that I looked over a few of those data structures yesterday just after lunch as part of some routine experiments, and... it's already 11am: "I.. yesterday I... I saw the array Bea. Uh..." (very hard)

The walls of the castle can only be breached by a hagfish with exactly seven gill apertures? Right sir, I'll get the siege eptatretus (impossible)